Passivation of inp solar cells using large area hexagonal-bn layers

ABSTRACT Surface passivation is crucial for many high-performance solid-state devices, especially solar cells. It has been proposed that 2D hexagonal boron nitride (hBN) films can provide

near-ideal passivation due to their wide bandgap, lack of dangling bonds, high dielectric constant, and easy transferability to a range of substrates without disturbing their bulk

properties. However, so far, the passivation of hBN has been studied for small areas, mainly because of its small sizes. Here, we report the passivation characteristics of wafer-scale, few

monolayers thick, hBN grown by metalorganic chemical vapor deposition. Using a recently reported ITO/i-InP/p+-InP solar cell structure, we show a significant improvement in solar cell

performance utilizing a few monolayers of hBN as the passivation layer. Interface defect density (at the hBN/i-InP) calculated using _C_–_V_ measurement was 2 × 1012 eV−1cm−2 and was found

comparable to several previously reported passivation layers. Thus, hBN may, in the future, be a possible candidate to achieve high-quality passivation. hBN-based passivation layers can

mainly be useful in cases where the growth of lattice-matched passivation layers is complicated, as in the case of thin-film vapor–liquid–solid and close-spaced vapor transport-based III–V

semiconductor growth techniques. SIMILAR CONTENT BEING VIEWED BY OTHERS HIGHLY PASSIVATED TOPCON BOTTOM CELLS FOR PEROVSKITE/SILICON TANDEM SOLAR CELLS Article Open access 30 September 2024

SILICON HETEROJUNCTION SOLAR CELLS WITH UP TO 26.81% EFFICIENCY ACHIEVED BY ELECTRICALLY OPTIMIZED NANOCRYSTALLINE-SILICON HOLE CONTACT LAYERS Article Open access 04 May 2023 A SILICON

CARBIDE-BASED HIGHLY TRANSPARENT PASSIVATING CONTACT FOR CRYSTALLINE SILICON SOLAR CELLS APPROACHING EFFICIENCIES OF 24% Article Open access 15 April 2021 INTRODUCTION At the semiconductor

surface, abrupt termination of the lattice can lead to defect states (called surface defect states) within the semiconductor bandgap. These surface defect states can significantly impair the

working of semiconductor devices, such as solar cells. Passivation is a method for reducing recombination through these surface defect states and is one of the most fundamental requirements

for achieving high-efficiency solar cells. In conventional III–V solar cells, epitaxially grown, lattice-matched III–V materials allowed very high-quality passivation1. However, most of

these lattice-matched wide bandgap III–Vs are only available for GaAs. Although high-efficiency InP solar cells have been realized using lattice-matched InGaAs and InAlAs passivation layers,

when used at the front, both of these materials have a sizeable parasitic absorption due to low bandgaps2,3,4,5. The lack of a wide bandgap passivation layer for InP has significantly

impeded its development compared to that of GaAs solar cells1. Other than epitaxial passivation layers, a substantial amount of work has also been done to find suitable non-epitaxial

passivation layers (mainly high _k_-dielectric), including but not limited to Al2O3, Gd2O3, LaF3, ZnO, ZnS, GaS, SiO2, MgO, Ta2O3, POx, Si3Nx, etc.1,6,7,8,9,10. Many of these dielectric

materials have been shown to function as suitable passivation layers; however, in most cases, controlled and reproducible formation of high quality and defect-free passivation layer remains

a challenging task11. Also, most of the work is reported for III–V transistors with very few reports on high-efficiency III–V solar cells, using dielectric passivation. On the other hand,

pristine 2D materials, in particular, hexagonal boron nitride (hBN) possess many desirable attributes, which makes them an ideal passivation layer. For instance, hBN and other 2D materials

lack dangling bond and can be transferred to a range of substrates without disturbing its bulk properties11,12. Also, hBN has a wide bandgap, high chemical and thermal stability, and high

dielectric constant (~2.4), which makes it ideal for surface passivation13. So far, the most pristine form of hBN is obtained through mechanical exfoliation from bulk crystals; however, the

lateral size of exfoliated flakes is limited to only a few tens of microns, which severely limits the scalability and large area application of hBN. Therefore, new methods such as chemical

vapor deposition (CVD), metalorganic chemical vapor deposition (MOCVD), and physical vapor deposition (e.g., DC magnetron sputtering) are being investigated to achieve large area hBN11,14.

hBN has recently been used as a passivation layer in silicon, GaAs, chalcogenide, organic, and 2D transition metal chalcogenides-based solar cells13,15,16,17,18,19. However, in most of these

cases, hBN used were very small in size, which is not practical for real solar cells application. In this study, we extend the application of hBN films for the passivation of InP-based

solar cells. Centimeter-size MOCVD-grown hBN films were transferred onto InP to study their passivation effect. We show that MOCVD-grown hBN can successfully passivate i-InP/p+-InP solar

cells to reduce the dark current, as well as the reverse leakage current by orders of magnitude, leading to a better performance of the device. The cells passivated with hBN has a _V_oc of

>800 mV, in comparison to 720 mV for unpassivated i-InP/p+-InP device. We also investigate the effect of hBN film thicknesses and demonstrate that while a thinner hBN is required to

achieve a sufficiently high _J_sc, a thicker hBN film leads to better passivation and higher _V_oc. External quantum efficiency (EQE) measurement of hBN passivated solar cells clearly show a

significantly improved (~20%) response in the blue region of the solar spectrum, thereby confirming the passivation of the solar cells in the presence of hBN monolayers. The passivation due

to hBN is further corroborated by double diode fitting and analysis of the dark IV curves. To gain further insight into the passivation effects of hBN, ultraviolet photoelectron

spectroscopy (UPS) and _C_–_V_ measurements were performed. Based on the analysis of our results, we postulate that the effectiveness of hBN as a passivation layer is a combined effect of

direct quantum mechanical tunneling and interface charge transfer. We also discuss the electron selectivity of hBN. RESULTS AND DISCUSSION HBN CHARACTERIZATION Before and after transferring

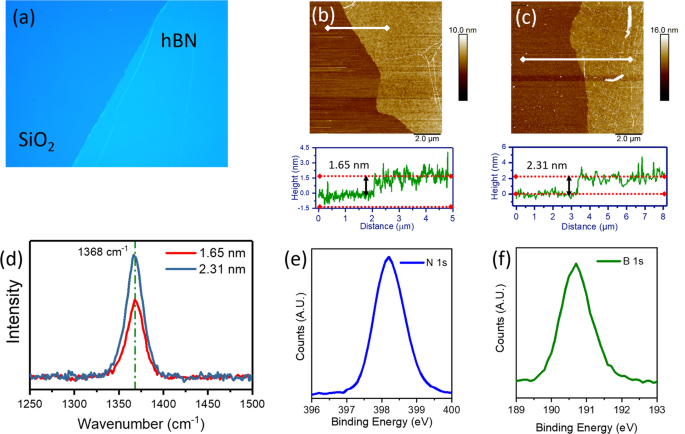
hBN on InP for device fabrication, we performed some basic characterizations of our hBN samples, as shown in Fig. 1. Figure 1a shows an optical micrograph of 7 ML hBN transferred onto

SiO2/Si substrate. The hBN covered region can be easily identified due to the difference in its refractive index with air. The thickness of the hBN film was measured using atomic force

microscope (AFM). Figure 1b, c respectively shows the thickness of 5 and 7 ML hBN films measured along the step edge, using AFM linescans. Raman spectra of 5 and 7 ML hBN films are shown in

Fig. 1d. The peak at 1368 cm−1 corresponds to the _E_2g vibrational mode of the _sp_2 bonded boron nitride20, and its intensity increase with film thickness. Furthermore, prior to device

fabrication, the successful transfer of hBN onto the InP substrate was also confirmed using x-ray photoelectron spectroscopy (XPS). Figure 1e, f shows the spectrum corresponding of B-1_s_

and N-1_s_ core levels at 190.6 and 398.2 eV, respectively, which confirmed the presence hBN on InP. SOLAR CELL IV AND EQE CHARACTERIZATION Figure 2 shows a 3D schematic and the

cross-sectional transmission electron microscopy (TEM) images of the solar cell. To assess the performance of hBN as a passivation layer, IV (light and dark) and quantum efficiency

measurements were performed on InP solar cells with and without hBN. For both 5 and 7 ML hBN passivated samples, we fabricated three devices, and the results were highly reproducible. Figure

3a shows IV characteristics of the hBN-InP solar cell @ 1 sun. It is quite evident from the figure that the introduction of hBN significantly improves both the _V_oc and _J_sc of the solar

cell, while also improving its fill factor. The maximum efficiency of 17.2% was obtained for 5 ML hBN with a _V_oc of 0.78 V, _J_sc of 29.4 mA cm−2 and a FF of 75.2%. The efficiency of InP

solar cell with 7 ML hBN was measured to be 15.7% with a _V_oc, _J_sc, and FF of 0.8 V, 27.1 mA cm−2, and 72.1%, respectively. This clearly shows that a thicker hBN gives a slightly higher

_V_oc, but significantly lower _J_sc suggesting that the hBN must not be too thick to achieve optimum performance of the device. In comparison to both 5 and 7 ML passivated InP solar cells,

the efficiency of unpassivated ITO/i-InP/p-InP remains significantly lower at 11.5% with a _V_oc of 0.72 V, _J_sc of 27.4 mA cm−2, and a FF of 58.6%. Here, we would like to mention that for

the MOCVD-grown hBN films, it has been shown that for the continuous coverage over the entire 2 in. sapphire substrate, a minimum growth time of 1 h was necessary20. This growth duration

resulted in hBN films with a thickness of 5 ML. Hence in the present study, hBN films thinner than 5 ML could not be obtained. Nonetheless, in the future, it will be exciting to see if

monolayers <5 ML can also provide sufficiently high passivation to achieve high efficiency. As expected, the lower _V_oc and _J_sc for unpassivated ITO/i-InP/p-InP solar cells are mainly

due to interface recombination and are consistent with previously reported results1,5. The EQE of a solar cell is a function of wavelength and is a direct measure of its _J_sc. It can be

written as a ratio of the number of photogenerated electrons collected to the number of photons of a given wavelength or energy incident onto the device: $$\begin{array}{*{20}{c}}

{{\mathrm{EQE}}\,\left( \lambda \right) = \,\frac{{I_{{\mathrm{ph}}}}}{{e.{\mathrm{{\Phi} }}_{\mathrm{p}}}} = \,\frac{{I_{{\mathrm{ph}}}}}{e}\,\left( {\frac{{h\upsilon

}}{{P_{{\mathrm{opt}}}}}} \right)} \end{array}$$ (1) where, _I_ph is the measured current in short-circuit condition when illuminated with light of frequency, \(\upsilon\) and optical power,

_P_opt_, e_ is the electronic charge, _h_ is the Planck’s constant, and \({\Phi} _{\mathrm{p}}\) is the photon flux. The EQE calculated is related to _J_sc of a solar cell using Eq. (2).

$$\begin{array}{*{20}{c}} {J_{\mathrm{sc}}\left( {\mathrm{EQE}} \right) = \mathop {\int }\nolimits_{\lambda 1}^{\lambda 2} \frac{{q\lambda }}{{hc}}\,\{ {\mathrm{EQE}}(\lambda

).\,{\mathrm{AM1.5G}}\left( \lambda \right)\} \,{\mathrm{d}}\lambda } \end{array}$$ (2) where _J_sc is the short-circuit current calculated for EQE measured between \(\lambda\)1 and

\(\lambda\)2. Using Eq. (2), the _J_sc calculated for the unpassivated device is 26.7 mA cm−2, whereas the _J_sc calculated for 5 ML and 7 ML passivated samples are 28.9 and 27.1 mA cm−2,

respectively. As expected, the calculated _J_sc is very close to the _J_sc measured using light generated IV curve shown in Fig. 3a. Moreover, because the different wavelength of light is

absorbed in different regions of the solar cell, wavelength-dependent EQE can provide information about optoelectronic losses in the different regions of the device. In particular, the

smaller wavelength light is absorbed in the topmost part of the absorber layer, and the quantum efficiency in this regime reflects the losses due to surface recombination. Figure 3b shows

measured EQE as a function of wavelength. It can be seen that there is a significant improvement in the EQE of the solar cell after 5 ML hBN passivation in the lower wavelength region, i.e.,

between 350 and 600 nm. An improvement of ~20% of EQE at the lower wavelength region for 5 ML hBN passivated samples in comparison to the unpassivated samples, indicate a significant

reduction in front surface recombination. Moreover, similar to 5 ML passivated samples, 7 ML passivated samples also show better EQE in the lower wavelength regime compared to unpassivated

samples, and a slightly higher EQE near 350 nm in comparison to 5 ML hBN passivated samples, suggesting slightly better passivation than 5 ML passivated samples. However, its overall quantum

efficiency is lower than that of 5 ML hBN due to reduced tunneling (as discussed later), with increased hBN thickness leading to a lower charge carrier collection, and _J_sc according to

Eqs.(1) and (2). Dark IV characteristics of a solar cell provide several useful information about device behavior in terms of recombination current, ideality factor, reverse leakage current,

and series and shunt resistances. The dark experimental IV of a solar cell can be fitted almost accurately using Eq. (3) below to derive parameters such as _J_01, _J_02, _n_1, _n_2, _R_s,

and _R_sh (ref. 10). $$J = \,J_{01}\,{\mathrm{exp}}\left[ {\frac{{q(V - JR_{\mathrm{s}})}}{{kT}}} \right] + \,J_{02}\,{\mathrm{exp}}\left[ {\frac{{q(V - JR_{\mathrm{s}})}}{{n_2kT}}} \right]

+ \,\frac{{V - JR_{\mathrm{s}}}}{{R_{{\mathrm{Shunt}}}}}$$ (3) In Eq. (3), the parameters, _J_01 and _J_02, respectively, denote dark current due to diffusion and recombination in the

depletion region, whereas, _n_1 and _n_2, denote the ideality factor of diodes, and _R_s and _R_sh denote the series and shunt resistances, respectively. Table 1 compares fitting parameters

of three different samples, and it is quite apparent that for all the samples, _J_01 is almost similar, which signifies that the recombination in bulk is identical in all the samples.

However, _J_02 is nearly an order of magnitude lower for hBN passivated samples compared to the unpassivated sample. This is primarily due to the passivation effect of hBN layer, which

modifies the carrier transport across i-InP/ITO interface (as discussed later), and reduces the recombination in the depletion regions. Also, all the solar cells have very high series and

low shunt resistance that is also reflected in the lower fill factor of the devices. Assuming a double diode model, the fill factor loss due to series (\({\mathrm{{\Delta}

}}{\mathrm{FF}}_{R_{\mathrm{s}}}\)) and shunt resistances (\({\mathrm{{\Delta} }}{\mathrm{FF}}_{R_{\mathrm{sh}}}\)) can be given by Eq. (4) (ref. 21). $${\mathrm{FF}}_0 = {\mathrm{FF}}

+\,\frac{J_{\mathrm{mpp}}^2 R_{\mathrm{s}}}{V_{{\mathrm{oc}}}J_{\mathrm{sc}}}+ \,\frac{\left( V_{{\mathrm{mpp}}} +

J_{{\mathrm{mpp}}}R_{\mathrm{s}}\right)^2}{R_{\mathrm{sh}}V_{\mathrm{oc}}J_{\mathrm{sc}}}$$ (4) $$\begin{array}{*{20}{c}} {{\Delta} {\mathrm{FF}}_{R_{\mathrm{s}}} =

\,\frac{{J_{{\mathrm{mpp}}}^2R_{\mathrm{s}}}}{{V_{{\mathrm{oc}}}J_{{\mathrm{sc}}}}}} \end{array}$$ (5) $${{\Delta} {\mathrm{FF}}_{R_{\mathrm{sh}}} = \,\frac{{\left( {V_{{\mathrm{mpp}}} +

J_{{\mathrm{mpp}}}R_{{{\mathrm{s}}}}} \right)^2}}{{R_{{\mathrm{sh}}}V_{{\mathrm{oc}}}J_{{\mathrm{sc}}}}}}$$ (6) In the above equation, FF_0_ denotes the fill factor of the resistance-free

cell and \({\mathrm{{\Delta} }}{\mathrm{FF}}_{R_{\mathrm{s}}}\) and \({\mathrm{{\Delta} }}{\mathrm{FF}}_{R_{\mathrm{sh}}}\), respectively, denote the fill factor loss due to series and shunt

resistances. Moreover, in addition to resistances, recombination in the depletion region can also lead to lower fill factor. To calculate loss due to depletion region recombination, we

firstly need to calculate an upper limit of fill factor for the cell, which can be given using an analytical equation proposed by Green22. $$\begin{array}{*{20}{c}} {{\mathrm{FF}}_{J_{01}} =

\,\frac{{v_{{\mathrm{oc}}} \, - \, \ln \left( {v_{{\mathrm{oc}}}} \ + \ {0.72} \right)}}{{v_{{\mathrm{oc}}} \ + \ 1}}} \end{array}$$ (7) where $$v_{{\mathrm{oc}}} =

\frac{q}{{nkT}}\,V_{{\mathrm{oc}}}$$ Combining Eqs. (7) and (4), the fill factor loss due to recombination in the depletion region is simply the difference between FF_J_01 and FF0. Moreover,

using Eq. 7, the upper limit of the fill factor (FFJ01) for both passivated and unpasssivated samples come out to be >85%. For 5 ML hBN passivated samples, the fill factor loss due to

series and shunt resistance comes out to be 4.1% and 0.1%, respectively, whereas the _J_02 recombination loss is ~6.3%. For 7 ML hBN passivated samples, with an increased thickness of hBN,

series resistance increases slightly, leading a slightly higher series resistance loss. However, as expected, fill factor loss due to shunt resistance and _J_02 recombination is almost

similar to 5 ML passivated samples. Most importantly, for unpasssivated samples, the most prominent fill factor loss mechanism is the recombination loss due to _J_02 current, which is

consistent with the results discussed earlier. UPS AND BAND ALIGNMENT To better understand the effect of hBN on the hBN/i-InP interface, we performed UPS measurements on ITO, i-InP, and

hBN/i-InP. The UPS spectra for samples with and without hBN are plotted in Fig. 4a, with binding energy (B.E.) on the _x_-axis and intensity on the _y_-axis. Before every measurement, the

instrument was calibrated against a standard silver sample and the valence band maxima (VBM) was calculated by extrapolating the valence band contribution to the B.E. axis. The work function

of the samples was calculated using the following equation: $$\begin{array}{*{20}{c}} {\phi = h\upsilon - \left( {E_{{\mathrm{cut - off}}} - E_{{\mathrm{Fermi}}}} \right)} \end{array}$$ (8)

where \(h\upsilon\) = 21.2 eV for He-I line, \(E_{{\mathrm{cut - off}}}\) is calculated by extrapolating the secondary electron onset to the _x_-axis, and \(E_{{\mathrm{Fermi}}}\) is the

corrected Fermi level. Because we calibrated the Fermi level before every measurement (using an Ag sample), for work function calculation, we assume \(E_{{\mathrm{Fermi}}}\) = 0. Using Eq.

(8), the work function of ITO and i-InP were calculated to be 4.8 and 5.1 eV, respectively. Surprisingly, there was no significant change in the work function of i-InP before and after hBN

transfer, as shown in Fig. 4a. However, there was a shift in VBM of i-InP after hBN transfer. Figure 4b shows the magnified valence band region of the i-InP, with and without hBN. The VBM of

i-InP moves by almost 0.27 eV toward the Fermi level in the presence of hBN. Based on UPS results, we draw a band diagram shown in Fig. 4c. It is quite evident in the band diagram that

there is an accumulation of electrons at the hBN/i-InP interface, which has an important consequence toward reducing the interface recombination and increasing the selectivity of electrons,

as discussed later in the passivation mechanisms section. PASSIVATION MECHANISM To further corroborate the observed passivation at the hBN/i-InP interface, high-frequency (100 kHz)

capacitance–voltage (_C_–_V_) measurements were performed on Au/ITO/hBN/i-InP/n-InP test structures, where ITO and Au were deposited through a shadow mask to form circular gate contacts.

Interface state density (_D_it) was extracted via the method of Terman (see Fig. 5)23, and similar energy-dependent interface state density was observed both 5 and 7 ML hBN passivated

samples, with a _D_it of 2–3 × 1012 eV−1 cm−2 at midgap. This compares favorably with reports for other passivating 3D thin films on InP, as compared in Table 2. However, unlike 3D

passivation layers, the passivation in the case of 2D hBN is not due to the passivation of dangling bonds. Based on previous reports, we postulate that 2D hBN passivates the surface defects

through the transfer of charges from surface defects states to hBN, which renders them electronically inactive and reduces electronically active interface defect density24. Although _C_–_V_

measurement provides evidence of hBN passivation, it does not provide the reasoning for thickness-dependent hBN passivation, as evidenced in IV and EQE measurements. To further explain

thickness-dependent hBN passivation and electron selectivity, we discuss the mechanism of charge carrier tunneling across hBN monolayers. Almost all quantum mechanical tunneling equation is

an extension of standard Fowler–Nordheim (FN) tunneling equation, which in its simplest form is given by: $$\begin{array}{*{20}{c}} {J_{{\mathrm{FN}}} = A \cdot \frac{{m_{{\mathrm{ox}}}^

\ast }}{{\phi _{\mathrm{b}}}} \cdot \left| {F_{{\mathrm{ox}}}} \right|^2 \cdot {\mathrm{exp}}\left( { - B \cdot \frac{{\left( {\phi _{\mathrm{b}}}

\right)^{\frac{3}{2}}}}{{F_{{\mathrm{ox}}}}}} \right)} \end{array}$$ (9) where, _A_, _B_, and _F_ox are given as follows: $$A = \,\frac{{q^3}}{{8\pi h}}$$ $$F_{{\mathrm{ox}}} =

\,\frac{{V_{{\mathrm{ox}}}}}{{t_{{\mathrm{ox}}}}}$$ $$B = \,\frac{{8\pi }}{3}\,\left( {\frac{{\sqrt {2 \cdot m_{ox}^ \ast } }}{{hq}}} \right)$$ In the above equation, _V_ox is the voltage

across the dielectric, \(\phi _{\mathrm{b}}\) is the effective barrier height, and \(m_{{\mathrm{ox}}}^ \ast\) is the effective electron mass in the dielectric layer (hBN). FN tunneling

equation mainly predicts that the tunneling current in the presence of a sufficiently high electric field, depends exponentially on the barrier height to the power 3/2. However, the FN

equation is only applicable to the triangular tunnel barrier, which mainly occurs when _V_ox > \(\phi _{\mathrm{b}}\). In the current case, we are mainly interested in the low voltage

regime, i.e., _V_ox < \(\phi _{\mathrm{b}}\), where most current flow across hBN through direct tunneling. Most recently, Lee and Hu proposed a modified FN model to calculate the direct

tunneling current (_J__i_) (Eq. 10)25. $$\begin{array}{*{20}{c}} {J_i = \frac{A}{{q\varepsilon _{\mathrm{ox}}\phi _{i,\mathrm{ox}}}} \cdot C\left( {V,V_{\mathrm{ox}},\,t_{\mathrm{ox}},\,\phi

_{i,\mathrm{ox}}} \right) \cdot {\mathrm{exp}}\left\{ { - \frac{{B\left( {\phi _{i,\mathrm{ox}}} \right)^{\frac{3}{2}}}}{{\left| {F_{\mathrm{ox}}} \right|}} \cdot \left[ {1 - \left( {1 -

\frac{{\left| {V_{\mathrm{ox}}} \right|}}{{\phi _{i,\mathrm{ox}}}}} \right)^{\frac{3}{2}}} \right]} \right\}} \end{array}$$ (10) The most significant difference between Eqs. (9) and (10) is

that they introduce a correction factor \(C\left( {V,V_{{\mathrm{ox}}},t_{{\mathrm{ox}}},\phi _{i,{\mathrm{ox}}}} \right)\) given by: $$\begin{array}{*{20}{c}} {C\left(

{V,V_{{\mathrm{ox}}},\,t_{{\mathrm{ox}}},\,\phi _{i,{\mathrm{ox}}}} \right) = {\mathrm{exp}}\left[ { - \frac{{20}}{{\phi _{i,{\mathrm{ox}}}}}\left( {\frac{{\left| {V_{{\mathrm{ox}}}} \right|

- \phi _{i,{\mathrm{ox}}}}}{{\phi _{i,{\mathrm{ox}}}}} + 1} \right)^{\alpha _{i,{\mathrm{ox}}}} \cdot \left( {1 - \frac{{V_{{\mathrm{ox}}}}}{{\phi _{i,{\mathrm{ox}}}}}} \right)} \right]

\cdot \frac{V}{{t_{{\mathrm{ox}}}}} \cdot N} \end{array}$$ (11) where, \(i\left( = {\mathrm{ECB}}\, {\mathrm{or}}\, {\mathrm{HVB}} \right)\) are the indexes to define tunneling of an

electron from the conduction band (_i_ _=_ ECB) or tunneling of holes from the valence band (_i_ _=_ HVB), _N_ is the density of carriers in the inversion or accumulation layer, and \(\alpha

_{i,{\mathrm{ox}}}\) is a fitting parameter. The exponential term associated with the correction factor accounts for secondary effects and only affects the curvature of tunneling

characteristics. But, one of the benefits of using Lee and Hu’s equation is that it directly correlates the tunneling current with the accumulation and inversion of carriers, and the

conduction and valence band offsets of i-InP/hBN (please see Fig. 6). Overall, Eqs. (9) and (10) respectively show that the tunneling current at any given voltage is inversely proportional

to the thickness of the dielectric layer and band offsets, and is directly proportional to the charge carrier density at the dielectric/semiconductor interface (hBN/i-InP). Therefore, in the

present scenario, a thinner hBN layer provides higher _J_sc compared to thicker hBN films. At the same time, with increased thickness of the hBN layer, the tunneling current of holes

(minority carrier) will also be reduced along with electrons, which will reduce the front contact recombination and lower the dark current, leading to a higher _V_oc for thicker hBN compared

to a thinner film. In addition to the passivation layer, hBN may also be acting as a selective contact. Previously, tunnel barriers have also been used as selective contacts26,27,28. For

solar cells using dielectric as selective contact, Peibst et al. showed that under first-order approximation, the contact resistance _ρ_c (determined by majority carrier flow), and the dark

current _J_0 (determined by minority carrier flow) are proportional to the transmission of electrons and holes25. Assuming a rectangular dielectric barrier (as shown in Fig. 6) and ignoring

the image charge effects, we can write the ratio for electrons and holes tunnel probabilities as follows26: $$\frac{{T_{\mathrm{e}}}}{{T_{\mathrm{h}}}}\, \propto \,\frac{{\exp \left\{ { -

t_{{\mathrm{ox}}}\sqrt {\frac{{2m_{{\mathrm{ox,e}}}^ \ast }}{{\hbar ^2}}\phi _{{\mathrm{ECB}}}} } \right\}}}{{\exp \left\{ { - t_{{\mathrm{ox}}}\sqrt {\frac{{2m_{{\mathrm{ox,h}}}^ \ast

}}{{\hbar ^2}}\phi _{{\mathrm{HVB}}}} } \right\}}}$$ (12) In the above equation, _T_e and _T_h are electrons and holes tunneling probabilities, respectively, _t_ox is the thickness of the

dielectric layer, \(m_{{\mathrm{ox,e}}}^ \ast\) and \(m_{{\mathrm{ox,h}}}^ \ast\) are the effective mass of electrons and holes in the dielectric, respectively. The mass of electrons and

holes in 2D hBN is similar at ~0.54 _m_0 (ref. 29). Therefore, for a given thickness of hBN, selectivity toward electrons or holes is solely decided by the barrier height. Therefore, when

there is an accumulation of electrons at the semiconductor/hBN interface (as in the current case), the barrier height for electron tunneling reduces, and hBN acts as an electron selective.

In contrast, when there is an accumulation of holes at the interface, it will become selective to holes. This is why hBN has previously been reported to act as both electron and hole

selective contacts depending on whether there is of electron or hole accumulation at the hBN/semiconductor interface30. METHODS HBN GROWTH AND CHARACTERIZATION The hBN films were grown on

commercially available 2 in. sapphire substrate using MOCVD, as described in ref. 14. The thickness of the films was controlled by varying the growth time. Although several different

thicknesses of hBN were investigated for passivation, to keep our discussion concise, here we only discuss the results from two different hBN films with a thickness of ~1.65 and ~2.15 nm

corresponding to five and seven monolayers of hBN (also referred to as 5 ML and 7 ML), respectively. For the passivation of the InP surface, cm-size hBN films were transferred from sapphire

to InP using the methods described in ref. 20. First, the sapphire substrate (with hBN) was broken into cm-size pieces. Thereafter, PMMA was spin-coated onto the hBN/sapphire substrate and

baked on a hot plate to remove excess solvent. The hBN layer was then floated in a 2% HF acid bath. The acid wetted the hBN–sapphire interface and etched the sapphire substrate laterally.

After a short time, the hBN layer separated from the sapphire and floated on the surface of the HF bath. Subsequently, the floating hBN–PMMA stack was fished out of the solution and washed

several times in deionized (DI) water to remove any residual HF. The hBN–PMMA was then carefully refloated on the surface of a DI water bath and then transferred onto the InP substrate. The

samples were allowed to dry under ambient for a few hours. Finally, PMMA was removed in acetone. Using the same process, the hBN–PMMA stack was also transferred on to a planar SiO2/Si

substrate for further characterization. After removing the PMMA layer, the transferred hBN films were characterized using AFM and Raman spectroscopy. Finally, cross-sectional TEM lamella was

prepared by focused ion beam milling using FEI’s Helios 600 NanoLab, and high-resolution TEM was performed with a JEOL 2100 F instrument. DEVICE FABRICATION AND CHARACTERIZATION A 3D

schematic and the cross-sectional TEM images of the solar cell are shown in Fig. 1. It consists of back ohmic contact of low resistance, a p+-InP base, an i-InP absorber layer, a hBN layer,

an ITO layer, and a metal contact over ITO. The device fabrication started with the epilayer growth of 2 microns thick i-InP on a p+-InP wafer. Next, a Zn/Au (20 nm/100 nm) metal contact

layer was evaporated on the back of the p+-InP substrate, followed by annealing at 400 °C in forming gas (5% H2:95% N2) for 40 min to create a low resistance back contact. Next, MOCVD-grown

hBN was transferred onto the InP substrate, as described above. The hBN adhered to the InP through van der Waals bonding and remains unaffected during further processing of the samples.

Thereafter, ITO was sputter-deposited on top of the hBN using conditions reported in ref. 5 Finally, a 500-nm thick silver is evaporated through a shadow mask to form the top contact. For

comparison, an InP solar cell without hBN layer was also fabricated in parallel under identical processing conditions. The device characterization started with the confirmation of the

successful transfer of hBN on InP using XPS. To understand the band bending at the interface of ITO/hBN/i-InP, UPS measurements were performed on i-InP, hBN/i-InP, and ITO. The IV

characteristics of the devices were obtained using an Oriel solar simulator and IV test station, under the 1-sun @ air mass 1.5 G (at 25 °C) and in the dark. Before every measurement, the

solar simulator was calibrated to 1-sun @ air mass 1.5 G (at 25 °C), using a standard test sample provided by the manufacturer. The dark IV curve was fitted using the double diode equation

to study the effect of hBN passivation. The algorithm used for fitting was based on the work of Suckow et al.21,22. The EQE of the sample was measured at room temperature using a quantum

efficiency setup from Oriel. Similar to the solar simulator, the EQE setup was calibrated using a commercially available test sample obtained from the manufacturer. DATA AVAILABILITY The

data used in this study are available upon request from the corresponding author V.R. REFERENCES * Raj, V., Tan, H. H. & Jagadish, C. Non-epitaxial carrier selective contacts for III-V

solar cells: a review. _Appl. Mater. Today_ 18, 100503 (2019). * Raj, K. Jain. InP solar cell with window layer. USA patent, US5322573 (1994). * Connolly, J. P. & Mencaraglia, D. in

_Materials Challenges: Inorganic Photovoltaic Solar Energy_, 209–246 (The Royal Society of Chemistry, Cambridge, 2015). * Mar, J. M. State-of-the-art of III-V solar cell fabrication

technologies, device designs and applications. in Advanced Photovoltaic Cell Design, Ch. 1, 1−8 (EN548, 2004). * Raj, V. et al. Indium phosphide based solar cell using ultra-thin ZnO as an

electron selective layer. _J. Phys. D Appl. Phys._ 51, 395301 (2018). Article  Google Scholar  * Robertson, J., Guo, Y. & Lin, L. Defect state passivation at III-V oxide interfaces for

complementary metal–oxide–semiconductor devices. _J. Appl. Phys._ 117, 112806 (2015). Article  Google Scholar  * Lin, L. & Robertson, J. Defect states at III-V semiconductor oxide

interfaces. _Appl. Phys. Lett._ 98, 082903 (2011). Article  Google Scholar  * Zhou, L. et al. Brief review of surface passivation on III-V semiconductor. _Crystals_ 8, 226 (2018). Article 

Google Scholar  * Raj, V., Fu, L., Tan, H. H. & Jagadish, C. Design principles for fabrication of InP-based radial junction nanowire solar cells using an electron selective contact.

_IEEE J. Photovolt._ 9, 980–991 (2019). Article  Google Scholar  * Raj, V., Vora, K., Fu, L., Tan, H. H. & Jagadish, C. High-efficiency solar cells from extremely low minority carrier

lifetime substrates using radial junction nanowire architecture. _ACS Nano_ 13, 12015–12023 (2019). Article  CAS  Google Scholar  * Hui, F. et al. On the use of two dimensional hexagonal

boron nitride as dielectric. _Microelectron. Eng._ 163, 119–133 (2016). Article  CAS  Google Scholar  * Cinzia C. F. W. _2D Materials for Nanoelectronics_ (CRC Press, Boca Raton, 2016). *

Shanmugam, M., Jacobs-Gedrim, R., Durcan, C. & Yu, B. 2D layered insulator hexagonal boron nitride enabled surface passivation in dye sensitized solar cells. _Nanoscale_ 5, 11275–11282

(2013). Article  CAS  Google Scholar  * Chugh, D. et al. Flow modulation epitaxy of hexagonal boron nitride. _2D Mater._ 5, 045018 (2018). Article  CAS  Google Scholar  * Cho, A.-J. &

Kwon, J.-Y. Hexagonal boron nitride for surface passivation of two-dimensional van der Waals heterojunction solar cells. _ACS Appl. Mater. Interfaces_ 11, 39765–39771 (2019). Article  CAS 

Google Scholar  * Kalita, G., Kobayashi, M., Shaarin, M. D., Mahyavanshi, R. D. & Tanemura, M. Schottky barrier diode characteristics of graphene-GaN heterojunction with hexagonal boron

nitride interfacial layer. _Phys. Status Solidi A_ 215, 1800089 (2018). Google Scholar  * Li, X. et al. Graphene/h-BN/GaAs sandwich diode as solar cell and photodetector. _Opt. Express_ 24,

134–145 (2016). Article  CAS  Google Scholar  * Li, Q., Zhou, Q., Shi, L., Chen, Q. & Wang, J. Recent advances in oxidation and degradation mechanisms of ultrathin 2D materials under

ambient conditions and their passivation strategies. _J. Mater. Chem. A_ 7, 4291–4312 (2019). Article  CAS  Google Scholar  * Shanmugam, M., Jain, N., Jacobs-Gedrim, R., Xu, Y. & Yu, B.

Layered insulator hexagonal boron nitride for surface passivation in quantum dot solar cell. _Appl. Phys. Lett._ 103, 243904 (2013). Article  Google Scholar  * Chugh, D., Jagadish, C. &

Tan, H. Large-area hexagonal boron nitride for surface enhanced raman spectroscopy. _Adv. Mater. Technol._ 4, 1900220 (2019). Article  CAS  Google Scholar  * Khanna, A. et al. A fill factor

loss analysis method for silicon wafer solar cells. _IEEE J. Photovolt._ 3, 1170–1177 (2013). Article  Google Scholar  * Green, M. A. Accuracy of analytical expressions for solar cell fill

factors. _Sol. Cells_ 7, 337–340 (1982). Article  CAS  Google Scholar  * Terman, L. M. An investigation of surface states at a silicon/silicon oxide interface employing metal-oxide-silicon

diodes. _Solid State Electron._ 5, 285–299 (1962). Article  CAS  Google Scholar  * Park, J. H. et al. Defect passivation of transition metal dichalcogenides via a charge transfer van der

Waals interface. _Sci. Adv._ 3, e1701661 (2017). Article  Google Scholar  * Wen-Chin, L. & Chenming, H. Modeling gate and substrate currents due to conduction- and valence-band electron

and hole tunneling [CMOS technology]. In _2000 Symposium on VLSI Technology. Digest of Technical Papers (Cat. No.00CH37104)_ 198–199 (IEEE, Honolulu, HI, 2000). * Peibst, R. et al. Working

principle of carrier selective poly-Si/c-Si junctions: is tunnelling the whole story? _Sol. Energy Mater. Sol._ 158, 60–67 (2016). Article  CAS  Google Scholar  * Rienäcker, M. et al.

Junction resistivity of carrier-selective polysilicon on oxide junctions and its impact on solar cell performance. _IEEE J. Photovolt._ 7, 11–18 (2017). Article  Google Scholar  * Wietler,

T. F. et al. Pinhole density and contact resistivity of carrier selective junctions with polycrystalline silicon on oxide. _Appl. Phys. Lett._ 110, 253902 (2017). Article  Google Scholar  *

Cao, X. K., Clubine, B., Edgar, J. H., Lin, J. Y. & Jiang, H. X. Two-dimensional excitons in three-dimensional hexagonal boron nitride. _Appl. Phys. Lett._ 103, 191106 (2013). Article 

Google Scholar  * Chu, D., Lee, Y. H. & Kim, E. K. Selective control of electron and hole tunneling in 2D assembly. _Sci. Adv._ 3, e1602726 (2017). Article  Google Scholar  Download

references ACKNOWLEDGEMENTS This work is supported by the Australian Research Council through the Discovery-Project grants. Access to the epitaxial growth and fabrication facilities is made

possible through the support of the Australian National Fabrication Facility, ACT Node. AUTHOR INFORMATION Author notes * These authors contributed equally: Vidur Raj, Dipankar Chugh.

AUTHORS AND AFFILIATIONS * Department of Electronic Materials Engineering, Research School of Physics, The Australian National University, Canberra, ACT, 2601, Australia Vidur Raj, Dipankar

Chugh, Hark Hoe Tan & Chennupati Jagadish * Research School of Electrical, Energy and Materials Engineering, ANU College of Engineering and Computer Science, The Australian National

University, Canberra, ACT, 2601, Australia Lachlan E. Black, M. M. Shehata & Daniel H. Macdonald * Australian National Fabrication Facility, Research School of Physics, The Australian

National University, Canberra, ACT, 2601, Australia Li Li * Centre for Advanced Microscopy, The Australian National University, Canberra, ACT, 2601, Australia Felipe Kremer * ARC Centre of

Excellence for Transformative Meta-Optical Systems, The Australian National University, Canberra, ACT, 2601, Australia Hark Hoe Tan & Chennupati Jagadish Authors * Vidur Raj View author

publications You can also search for this author inPubMed Google Scholar * Dipankar Chugh View author publications You can also search for this author inPubMed Google Scholar * Lachlan E.

Black View author publications You can also search for this author inPubMed Google Scholar * M. M. Shehata View author publications You can also search for this author inPubMed Google

Scholar * Li Li View author publications You can also search for this author inPubMed Google Scholar * Felipe Kremer View author publications You can also search for this author inPubMed 

Google Scholar * Daniel H. Macdonald View author publications You can also search for this author inPubMed Google Scholar * Hark Hoe Tan View author publications You can also search for this

author inPubMed Google Scholar * Chennupati Jagadish View author publications You can also search for this author inPubMed Google Scholar CONTRIBUTIONS L.E.B. and M.M.S. performed CV

measurement and analysis. L.L. and F.K. performed FIB and TEM, respectively. D.H.M., H.H.T., and C.J. supervised the project. V.R. wrote the manuscript with contribution from all the

authors. All authors have given approval to the final version of the manuscript. CORRESPONDING AUTHORS Correspondence to Vidur Raj or Hark Hoe Tan. ETHICS DECLARATIONS COMPETING INTERESTS

The authors declare no competing interests. ADDITIONAL INFORMATION PUBLISHER’S NOTE Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional

affiliations. RIGHTS AND PERMISSIONS OPEN ACCESS This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution

and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if

changes were made. The images or other third party material in this article are included in the article’s Creative Commons license, unless indicated otherwise in a credit line to the

material. If material is not included in the article’s Creative Commons license and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to

obtain permission directly from the copyright holder. To view a copy of this license, visit http://creativecommons.org/licenses/by/4.0/. Reprints and permissions ABOUT THIS ARTICLE CITE THIS

ARTICLE Raj, V., Chugh, D., Black, L.E. _et al._ Passivation of InP solar cells using large area hexagonal-BN layers. _npj 2D Mater Appl_ 5, 12 (2021).

https://doi.org/10.1038/s41699-020-00192-y Download citation * Received: 19 March 2020 * Accepted: 02 December 2020 * Published: 08 January 2021 * DOI:

https://doi.org/10.1038/s41699-020-00192-y SHARE THIS ARTICLE Anyone you share the following link with will be able to read this content: Get shareable link Sorry, a shareable link is not

currently available for this article. Copy to clipboard Provided by the Springer Nature SharedIt content-sharing initiative